Marcus du Sautoy, publiciser of understanding of science, was recently in Bombay to talk up his latest book The Number Mysteries: A Mathematical Odyssey Through Everyday Life. He visited the Tata Institute of Fundamental Research, that bastion of world-class research in physics and mathematics and biology, where he gave one of his stirring lectures that have made him so popular in high schools and on television everywhere.
Now, academics are a singularly bitchy community, and the mathematicians were upset that du Sautoy, a mathematician, was pitching his talk at the level of a middle-school student, or possibly a bright biologist. The biologists were very pleased, however: they could actually understand his mathematics! He spoke of his twin passions - football and numbers - and, self-deprecating as any Englishman, poked fun at his own club team's abysmal performance. The reason for it, he said, was that their shirt numbers were so humdrum, resulting in their always featuring at the bottom of their league tables.
One year, they decided to assign themselves prime numbers, and they ended up runners-up. Exhilarated by this deep understanding into sports performance, they continued with the prime numbers the next year. Unfortunately, they were beaten by nearly every other team they faced.
Continuing in the vein of prime numbers, he talked about David Beckham's number 23 shirt, and, of course, Michael Jordan's career with the same number. He could not, he said, think of any reason why the number 23, a prime, should be interesting.
One of the mathematicians in the audience felt he should pipe up at that moment to give two reasons for the beauty of the number 23. But he didn't. Instead, he explained it all to me.
If you take the set Q(p) of all numbers of the form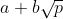 
, where a, b are rationals, p is a square-free number (i.e., a number without any square divisors), you can show that the set forms what is known as a field. Within this field, you can construct a subset that corresponds to it as the integers do to the set of rationals. The integers have a property called Unique Factorisation. In other words, any integer can be decomposed into a product of primes, and this product is unique. For example, 23 is a prime, and so its unique factorisation remains itself; but 24 = 2 x 2 x 2 x 3, and this is the only way to decompose 24 into primes.
It turns out that there are some values of p such that the set 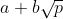has a subset with the unique factorisation property. On the other hand, there are other values of p for which the factorisation is not unique. The metric of how badly unique factorisation fails is given by a mathematical term called the class number of the field. For fields with unique factorisation subsets, the class number is 1.
It turns out that for small negative values of p, the corresponding class numbers are 1 or 2, or occasionally 4. In fact, it is known exactly when the class number will be even. But Q(-23), the set of numbers of the form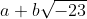 
has class number 3. This is the first time class number 3 appears. Why does it turn up at -23? What's so special about -23?
(You could ask, though - why not? After all, if the class number can take whatever value (and I'm not saying it does. I'm not saying it doesn't, either. Some mathematician may correct me if they feel like it), it will have to happen at some number. So why at -23?)
The next reason for the surprising qualities of the number 23 has to do with Ramanujan's tau function. We can write one particular expression of a product of powers of q as a sum of powers of q multiplied by the tau function. For example:
That tau function has some interesting properties:
And a chap called Pierre Deligne won the Fields medal for (among others) his proof of the following property, which was, according to my pal, conjectured by Ramanujan:
Lehmer conjectured that tau(n) is never zero. It is known, though, that tau(n) can be zero modulo a prime. But the funky thing, where the number 23 gets involved, is in the following property:
tau(p) = 0 modulo 23 for half the primes p.
(It is even known which primes p, but we needn't go into that now.)
So how about that? There's a funky function, which when fed half the prime numbers, results in a multiple of 23.
In fact, as it turns out, this property of the number 23 is closely related to the earlier property we mentioned, namely that Q(-23) has class number 3! Deep connections in the mathematical terra firma, and all that...
I'm not sure if this has anything to do with the fact that in the equation above introducing t(n) we took powers of 24. You know, 24 being right next to 23, etc. My pal wasn't sure if there was any connection. He didn't study smaller powers than 24, he said, because they didn't result in modular forms. 24 was the first power of any interest at all to him, and it had this neat property.
Too bad he didn't mention all this to Marcus du Sautoy. Perhaps the good professor can address these and other interesting facts about the number 23 at a future lecture?
2 comments:
I will send you a paper on the ancient kerala school that should prove interesting thaough you would have heard much about those things already...
Thanks! I got Kim Plofker's recent book on Indian math, and need to go through it shortly.
Post a Comment